The Pythagorean Theorem I
By any standard, the Pythagorean theorem is the most well-known theorem in all of mathematics. It shows up, directly or in disguise, in almost every branch of it, pure or applied. It is also a record breaker in terms of the number of proofs it has generated since Pythagoras allegedly proved it around 500 BCE. And it is the one theorem that almost everyone can remember from his or her high school geometry class.
Most of us remember the Pythagorean theorem by its famous equation, a2 + b2 = c2. The Greeks, however, thought of it in purely geometric terms, as a relationship between areas. This is how Euclid stated it: in all right-angled triangles the square on the side subtending the right angle is equal to the squares on the sides containing the right angle. That is, the area of the square built on the hypotenuse (“the side subtending the right angle”) is equal to the combined area of the squares built on the other two sides.
Pythagoras of Samos (ca. 580–ca. 500 BCE) may have been the first to prove the theorem that made his name immortal, but he was not the first to discover it: the Babylonians, and possibly the Chinese, knew it at least twelve hundred years before him, as is clear from several clay tablets discovered in Mesopotamia. Furthermore, if indeed he had a proof, it is lost to us. The Pythagoreans did not leave any written records of their discoveries, so we can only speculate what demonstration he gave. There is, however, an old tradition that ascribes to him what became known as the Chinese proof, so called because it appeared in an ancient Chinese text dating from the Han dynasty (206 BCE – 220 CE; see figure 5.1). It is perhaps the simplest of the more than 400 proofs that have been given over the centuries.
The Chinese proof is by dissection. Inside square ABCD (figure 5.2) inscribe a smaller, tilted square KLMN, as shown in (a). This leaves four congruent right triangles (shaded in the figure). By reassembling these triangles as in (b), we see that the remaining (unshaded) area is the sum of the areas of squares 1 and 2, that is, the squares built on the sides of each of the right triangles.
Elisha Scott Loomis (1852–1940), a high school principal and mathematics teacher from Ohio, collected all the proofs known to him in a classic book, The Pythagorean Proposition (first published in 1927, with a second edition in 1940, the year of his death). In it you can find a proof attributed to Leonardo da Vinci, another by James A. Garfield, who would become the twentieth president of the United States, and yet another by Ann Condit, a 16-year-old high school student from South Bend, Indiana. And of course, there is the most famous proof of them all: Euclid’s proof. We will look at it in the next chapter.
Our illustration (plate 5) shows a 45-45-90-degree triangle with squares—or what looks like squares—built on its sides and on the hypotenuse. But wait! Something strange seems to be going on: 52 + 52 = 72, or 25 + 25 = 49! Did anything go wrong? Do we see here an optical illusion? Not really: the illustration is, after all, an artistic rendition of the Pythagorean theorem, not the theorem itself; as such it is not bound by the laws of mathematics. To quote the American artist Josef Albers (1888–1976): “In science, one plus one is always two; in art it can also be three or more.”
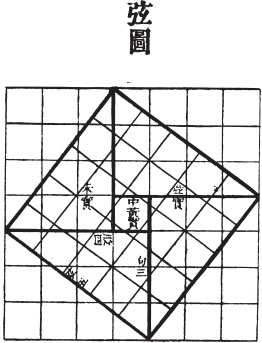
Figure 5.1. Joseph Needham, Science and Civilisation in China, courtesy of Cambridge University Press, Cambridge, UK.

Figure 5.2

