One Theorem, Three Proofs
Theorem 13 of Book VI of Euclid tells us how to find the geometric mean (the mean proportion, as the Greeks called it) of two line segments. In essence, it says that in a right triangle, the altitude h divides the hypotenuse into two segments m and n such that h/m = n/h. From this it follows that h2 = mn, so that h is the geometric mean of m and n. Plate 13 illustrates this for m = 9, n = 4, and h = 6.
We offer here three quite different proofs, with the question in mind, which of them is the simplest? Let the triangle be ABC, with the right angle at C (figure 13.1). From C drop the altitude ![]() = h to the hypotenuse, dividing it into segments
= h to the hypotenuse, dividing it into segments ![]() = m and
= m and ![]() = n. The first proof—the one found in most geometry textbooks—relies on the similarity of triangles ADC and CDB, since they share a right angle at D and equal angles ∠DAC and ∠DCB. Thus,
= n. The first proof—the one found in most geometry textbooks—relies on the similarity of triangles ADC and CDB, since they share a right angle at D and equal angles ∠DAC and ∠DCB. Thus, ![]() (as before, all line segments are nondirectional, so that
(as before, all line segments are nondirectional, so that ![]() ).
).
The second proof is a direct consequence of Thales’s theorem (or rather its converse; see chapter 1, note 1) and Euclid III 35 (chapter 11). Inscribe triangle ABC in a circle with diameter AB, as in figure 13.2. This circle passes through C and through its mirror image C′ when reflected in the diameter. Hence m · n = h · h = h2—QED.
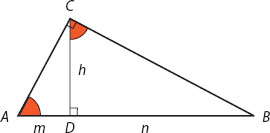
Figure 13.1

Figure 13.2
The third proof relies on a comparison of areas. We turn triangle ADC counterclockwise through 90° about D to get the skewed butterfly-like figure BCDA′C′D (figure 13.3). Note that angles ∠C′A′D and ∠BCD are equal, and therefore lines BC and A′C′ are parallel, with CA′ acting as a transversal (Euclid I 27). We now construct square CD′C′D with area h2 and rectangle A′B′BD with area mn, and divide each into two halves by diagonals C′C and A′B. Triangles C′A′B and C′A′C have the same area, since their vertices B and C lie on a line parallel to the base A′C′ (Euclid I 38). From each of these triangles subtract triangle A′DC′, resulting in triangles C′DC and A′DB having the same area. But triangle C′DC has half the area of square CD′C′D, and triangle A′DB has half the area of rectangle A′B′BD. Thus, h2 = mn.
We return now to the question we posed at the beginning of this chapter: which of the three proofs is the simplest? Judging by their length, the second proof is the obvious winner, requiring just four lines of explanatory text. But length is only one criterion of what constitutes a simple proof. Another criterion—and arguably a more important one—is how many previously established propositions the theorem in question is directly based upon. And this puts the third proof up front: it rests on just two earlier propositions—Euclid I 27 and I 38. Moreover, it is in line with the Greek interpretation of a product as the area of a rectangle, and in this sense it echoes Euclid’s famous proof of the Pythagorean theorem (Euclid I 47).
Of course, even this criterion is not entirely foolproof, as each of the two theorems on which the last proof relies is itself resting on several earlier theorems. The complete ancestry of a theorem may be long and arduous, making any absolute judgment of which proof is the simplest almost impossible. Ultimately, simplicity is in the eyes of the beholder.

Figure 13.3

