Sierpinski’s Triangle
Waclaw Franciszek Sierpinski (1882–1969) was born in Warsaw. He entered the University of Warsaw in 1899, graduating in 1904; four years later he was appointed to the University of Lvov. He became interested in set theory after reading about a theorem that allows for a seemingly impossible situation. We learn in analytic geometry that any point in the plane can be uniquely specified by two numbers, its x- and y-coordinates. This has been the rock foundation of analysis ever since René Descartes invented his analytic geometry in 1637. Not so, said the theorem that Sierpinski came across: one number suffices. Intrigued, he wrote to the article’s author, Tadeusz Banachiewicz, asking for an explanation. He got a reply of a single word: “Cantor,” referring to the creator of modern set theory, Georg Cantor, the subject of our last chapter. Becoming hooked on the subject, Sierpinski started studying it and gave the first-ever university course devoted entirely to set theory. The topic would occupy him for the rest of his life.1
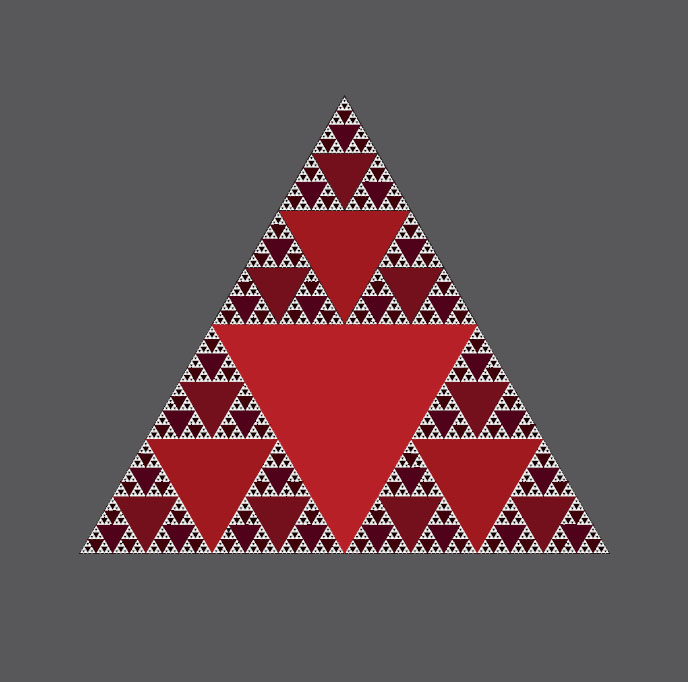
As an offshoot of his research, Sierpinski in 1915 came up with a seemingly impossible geometric configuration: a triangle-like figure whose area is zero. Start with an equilateral triangle (figure 50.1), and remove from it the small central triangle formed by connecting the midpoints of the sides (shown in white in the figure). Now repeat the process with the three remaining black triangles, then repeat it again and again … forever. In the limit, what will be the area of all the black triangles?
Taking the area of the initial triangle to be 1, each of the three smaller black triangles has an area ¼, so their combined area is 3 × ¼ = ¾. In the next step we have nine black triangles, each with area z, making the total area 9 × z = (¾)2. Continuing in this manner, the black areas follow the progression 1, ¾, (¾)2, (¾)3, …, a geometric progression with the common ratio ¾. Since this ratio is less than 1, the terms of the progression tend to 0 as n → ∞. So, eventually the original black triangle will become empty, despite the fact that at each stage we removed only ¼ of each black area.

Figure 50.1
On the other hand, the perimeters of the black triangles follow the sequence 3, >x, XMv, <Z,, …, a geometric progression with the common ratio Cx. Since this ratio is greater than 1, the terms grow without bound as we keep removing more and more triangles, making the limiting perimeter infinite. That is to say, the limiting shape—known as Sierpinski’s triangle—has zero area but infinite length! It shows again that when infinity comes into play, strange things always lurk around the corner.2
While we cannot go to infinity in the actual world, we can get pretty close with a computer, stopping only when the resolution reaches its limit at the pixel level. Plate 50 shows the seventh stage of Sierpinski’s triangle.
NOTES:
1. This biographical sketch is based on the article “Waclaw Sierpinski” by John J. O’Connor and Edmund F. Robertson in The MacTutor History of Mathematics Archive (on the Web), listed in the bibliography.
2. The formation of Sierpinski’s triangle can be viewed as an animation at http://en.wikipedia.org/wiki/Sierpinski_triangle.